Frequently Asked Questions

How is your approach to the physics of lasers different than previous random laser studies and/or standard laser theory?

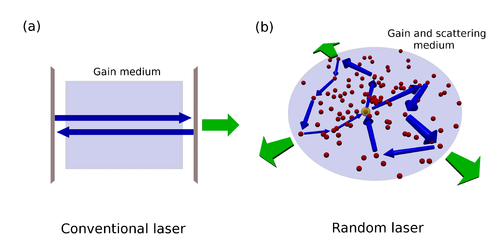
Our approach to laser theory is different from all previous approaches we are aware of in that we formulate a time-independent theory of the steady-state of a laser which is "ab initio": given the laser resonator and simple properties of the gain medium we predict the output power as a function of the pump, the number of lasing modes, the lasing frequencies and the spatial dependence of the electric field inside and outside the resonator. Previous theories were based either on time-dependent simulations or on the assumption that passive cavity resonances (often assuming perfectly reflecting mirrors and no leakage) describe the lasing modes. For random lasers, the time-dependent simulations are difficult to do (but there are some) and hard to interpret, and they don't answer the question: what is the nature of the lasing modes in a random laser. The passive cavity approach fails spectacularly. As we show in our paper, the lasing modes have little to do with the passive cavity resonances.

Could you briefly describe how your results were obtained?

We implemented our analytic formulation in a detailed code, the first of its kind. It solves the self-consistent equation we have derived for the lasing state and outputs all the physical quantities. The results are much easier to obtain and to interpret than those of time-dependent simulations. Ultimately, we hope that such a code could be used as a design tool for micro- and nano-lasers.

What are the next steps in your studies in this research field?

We are currently making detailed quantitative tests of our approach, by comparing it to full simulations of the Maxwell-Bloch lasing equations. Initial results are positive and we hope to prove that our approach can replace these simulations in many situations. This will make arbitrarily complex laser systems accessible to theoretical design, whereas up to now the field of microlasers is approached very empirically.

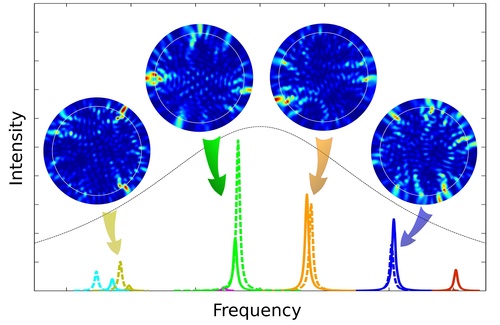
In addition, we have just begun to understand random lasers using our approach and there is a lot of new questions that our findings have spawned. First and foremost, we want to characterize and understand fully the statistical properties of random lasers operating in various regimes, and see how they relate to other disordered photonic or electronic systems. Ultimately, we hope their statistical properties can be predicted fully analytically and further experiments can be done to confirm the theory.

Finally, our theory is an improved version of what is called "semiclassical laser theory" in the textbooks; that means that we do not treat the electromagnetic field quantum-mechanically. All of the noise and coherence properties of lasers require a quantum treatment; it will be fascinating to see if our new insights into the non-linear lasing states can be generalized and used to improve our understanding of the quantum properties of novel and complex lasers.

What previously unresolved problems or questions, if any, can now be attempted using your new understanding of random laser physics?

For many of the proposed applications of random lasers, it is important to know how stable the lasing frequencies are over time and with respect to different pump conditions. Our theory is able to provide answers to such questions. Also the interaction between laser modes ("spatial hole burning") and its consequences on lasing thresholds and output intensities are fully accessible by our theoretical framework. Finally, there is the issue of Anderson localized random lasers versus diffusive random lasers, which makes a distinction between the lasing properties based on the strength of scattering provided by the sub-wavelength particles. The spatial distribution of modes as well as the distribution of frequencies and lifetimes in these two regimes are expected to be drastically different. At least this is the expectation from the linear scattering properties. It remains to be seen how the non-linearity and the resulting interactions modify this expectation in the steady state.

What is the significance of your results for the laser physics community, and how will your results impact future research in the area?

Our approach replaces the passive cavity resonance picture, and works in the highly non-linear regime of lasing well above threshold. Also it treats the openness of the laser cavity system exactly in terms of functions we term “constant flux states”. Therefore, in our most optimistic scenario, this approach would become the standard way to solve and understand the semiclassical laser theory in textbooks. Ultimately it becomes part of the answer to the question: how does a laser work?

Future research in designing novel micro and nanolasers can benefit from this approach, and we are implementing some of these ideas already with experimental collaborators to improve, e.g. power output, directional emission, etc. These applications are in general not related random lasers.

Finally, our results specifically on random lasing may have important general implications e.g. for the physics of disordered and (wave-)chaotic systems in a non-linear medium. It is now clear from our work that a proper description of the statistical properties of random lasing, for instance, has to take into account the strong modal interactions that we find. In some respects, this problem is the analogue of the physics of interacting electrons in a disordered landscape -- a cornerstone problem of condensed matter physics -- for a bosonic field.